dynamical Chaos in Planetary Systems
The question of whether or not the solar system is bound to unravel gravitationally dates back to the very origins of physical science and Newton himself. Today, it is largely agreed upon that the solar system did indeed suffer a transient orbital instability early in its lifetime (Tsiganis et al 2005; Batygin & Brown 2010; Batygin et al 2011; 2012), before settling into its calm present-day state.
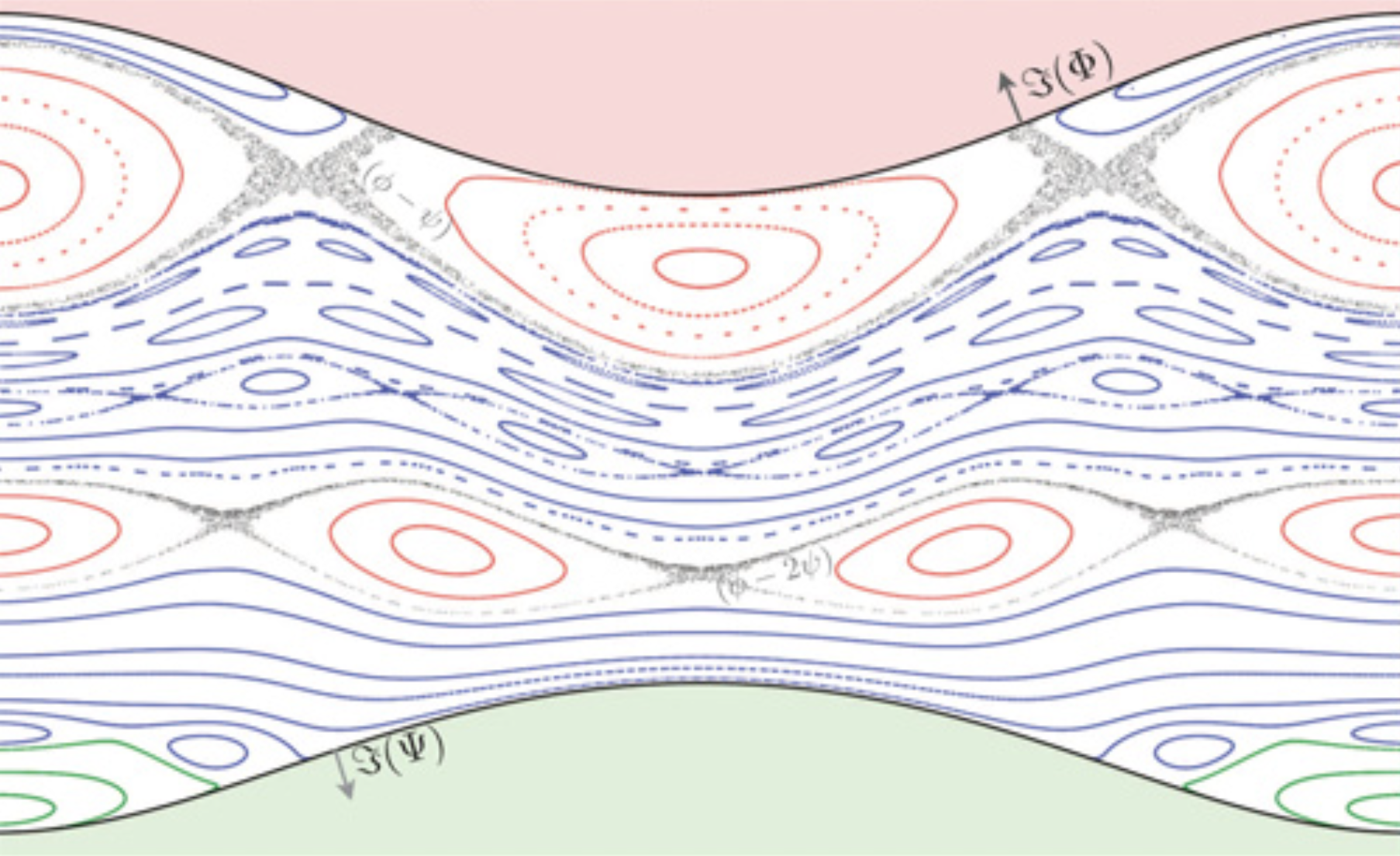
Intriguingly, the possibility of orbital rearrangement within the solar system is not confined to its earliest epochs. In spite of ‘proofs’ of eternal dynamical stability presented by Laplace (1775), Lagrange (1778), and Gauss (1809), large-scale numerical simulations of the solar system’s long-term evolution have revealed that the solar system exhibits chaotic behavior on multi-million year timescales, and that the there exists a ~1% chance that Mercury will eject from the solar system within the Sun’s remaining main sequence lifetime (Laskar 2008; Batygin & Laughlin 2008; Laskar & Gastineau 2009). In Batygin et al. (2015a), we presented a purely analytical description of the solar system’s instability, providing a qualitative understanding the solar system’s long-term chaotic behavior and delivering the final nail into the coffin of the belief in the solar system’s enduring immutability.
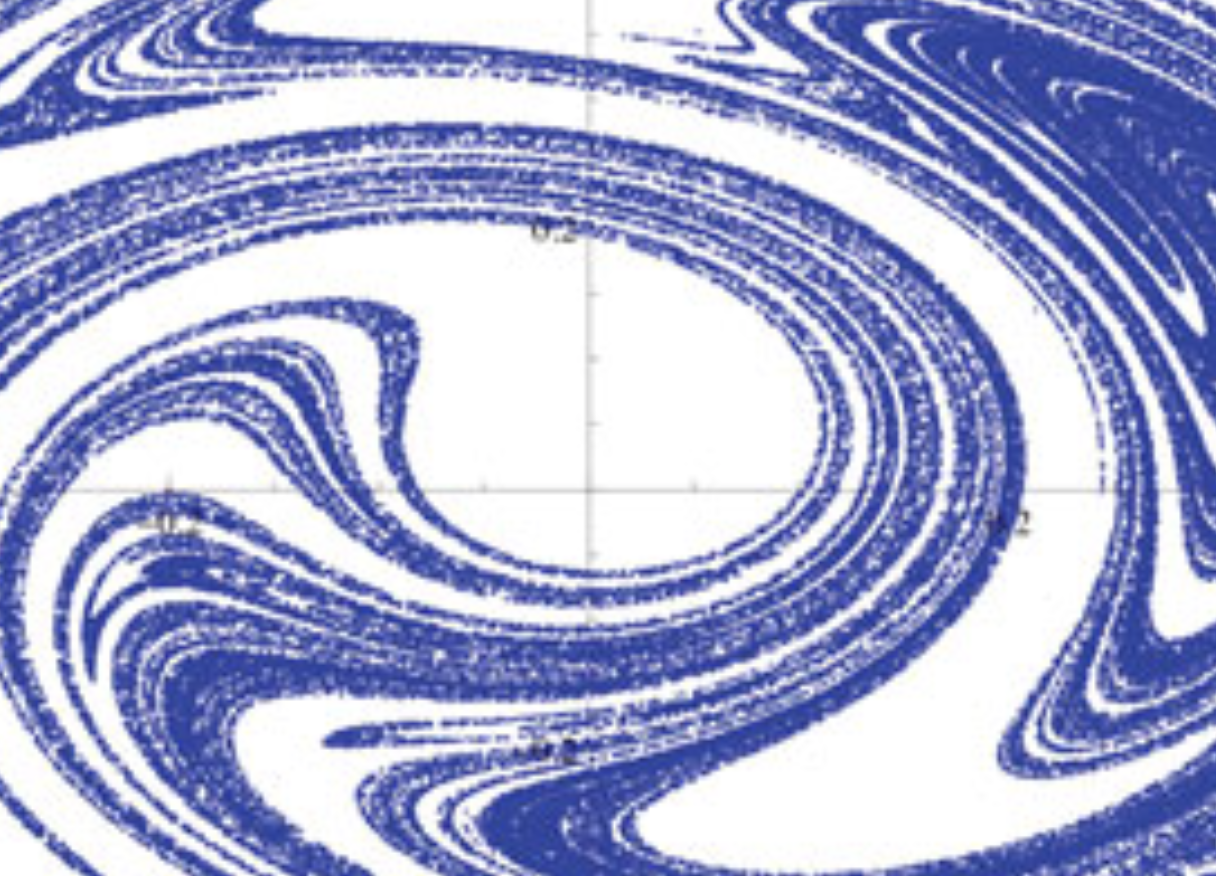
Chaotic evolution is by no means special to the solar system. The M-dwarf GJ876 (the closest known star to harbor a multi-planetary system) is encircled by four planets, three of which are locked in Laplace-type orbital resonance. In Batygin et al. (2015b), we showed that the evolution of this resonance is far more chaotic than previously thought, and is characterized by a Lyapunov time (roughly speaking, the orbital decoherence time) of a mere 7 years, constituting a rare example of observable astrophysical chaos.